Interpretation problems of impedance measurements on time variant systems Battery & Corrosion – Application Note 55
Latest updated: May 28, 2024Abstract
Electrochemical systems like a battery in operation or a corroding electrode are rarely time-invariant. Impedance graphs obtained on time-variant systems can often mislead to a wrong interpretation and analysis when using the equivalent electrical circuit. This application note describes two examples of such a mislead interpretation using a commonly used electrical equivalent circuit with a variable resistor.
Introduction
Application note #9 [1] deals with impedance measurements performed on linear (and non-linear) time-invariant systems. Electrochemical systems like a battery in operation or a corroding electrode are rarely time-invariant. Impedance graphs obtained on time-variant systems can often mislead to incorrect interpretation and analysis when using equivalent electrical circuit. This application note describes two examples of such misleading interpretations using a commonly used electrical equivalent circuit with a variable resistor.
Impedance Diagram for Time-Variant System
R1+R2(t)/C2 Circuit with Increasing R2
The time evolution of systems, such as operating batteries or corroding electrodes, is not known a priori and hence cannot be predicted.

Figure 1: R1+R2(t)/C2 circuit.
Let us consider the R1+R2(t)/C2 circuit [2] (ZFit was used to graphically code the circuit) shown in Fig. 1 and assume that the resistance R2 increases with time according to the following relationship:
$$R2(t)=R2_0 + kt^2\tag{1}$$
The impedance was calculated for a measurement carried out with one period of sinusoidal signal. The impedance is calculated at the frequency fn using the R2 resistance value at time t given by
$$t(f_n)=\sum^n_{i=1}t(f_i)=\sum^n_{i=1}\frac{1}{(f_i)}\tag{2}$$
where t(fi) is the duration of the measurement of one point on the diagram, i.e. the inverse of the measurement frequency [3].

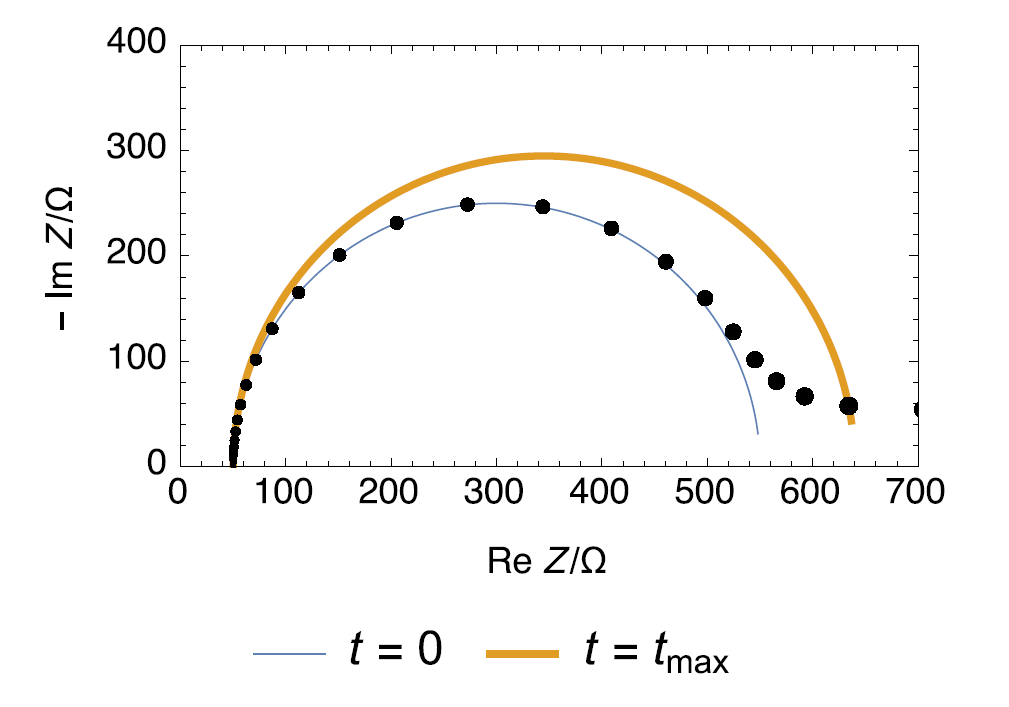
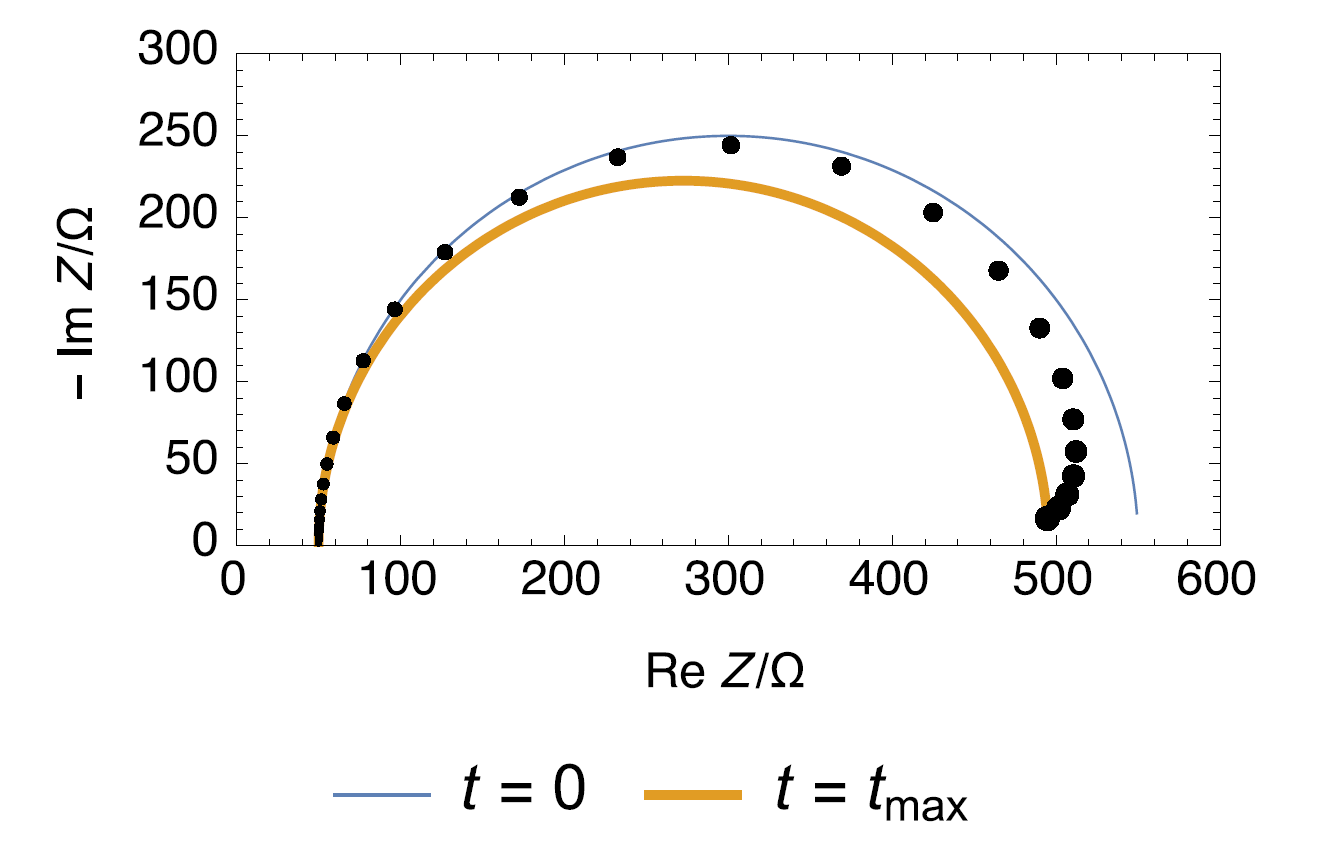
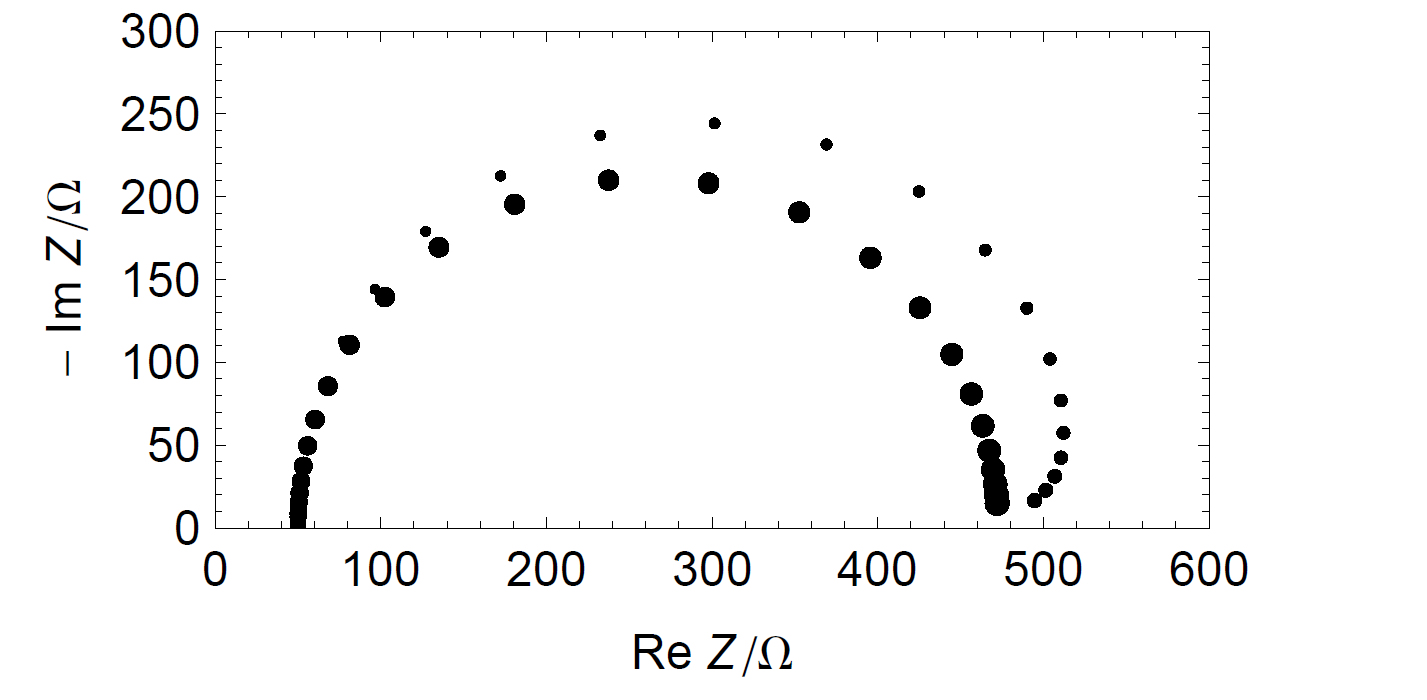
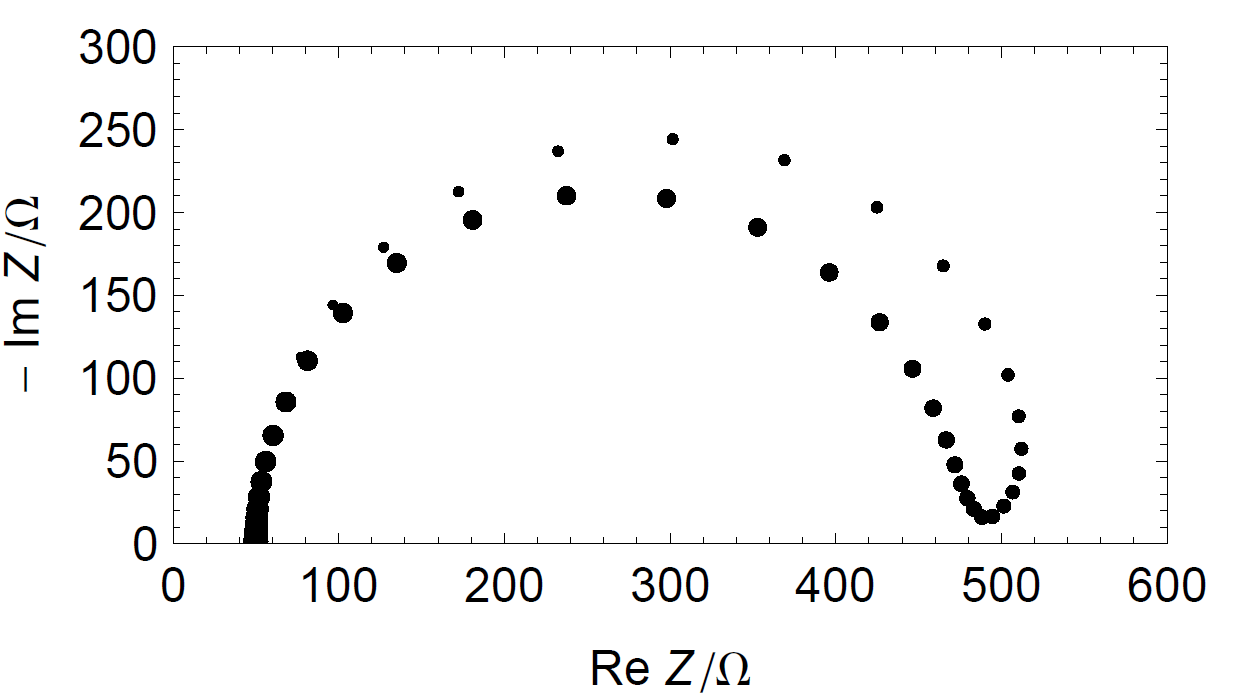
Figure 2: Instantaneous Nyquist impedance diagrams (t = 0 and t = tmax) of the circuit R1+R2(t)/C2 (R1 = 50 Ω, R20 = 500 Ω, k = 10–5 Ω s–2, C2 = 2.10–2 F) and simulation of the measured diagram (•). The dot size increases with increasing time.

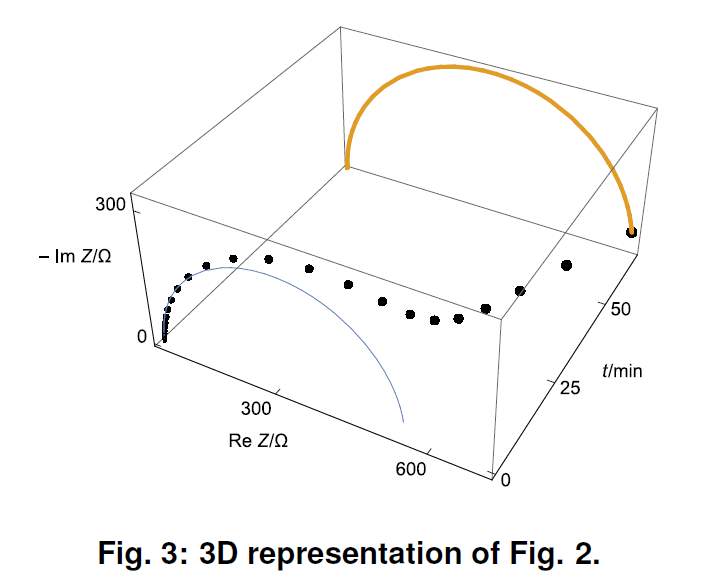
Figure 3: 3D representation of Fig. 2.
The frequency sweep is performed from fmax ! fmin with fmax = 10 Hz and fmin = 10−3 Hz. All frequencies are logarithmically distributed over 8 points per decade. The parameters used for R1, R2 and C2 are described in the caption of Fig. 2.
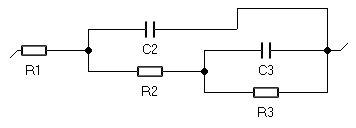
The simulated impedance diagram in Figs. 2 and 3 show the beginning of a low frequency arc. The impedance of the circuit described in Fig. 4 can show a time-invariant Nyquist graph similar to the time-variant Nyquist graph of the circuit shown in Fig. 1.

Figure 4: R1+C2/(R2+C3/R3) circuit.
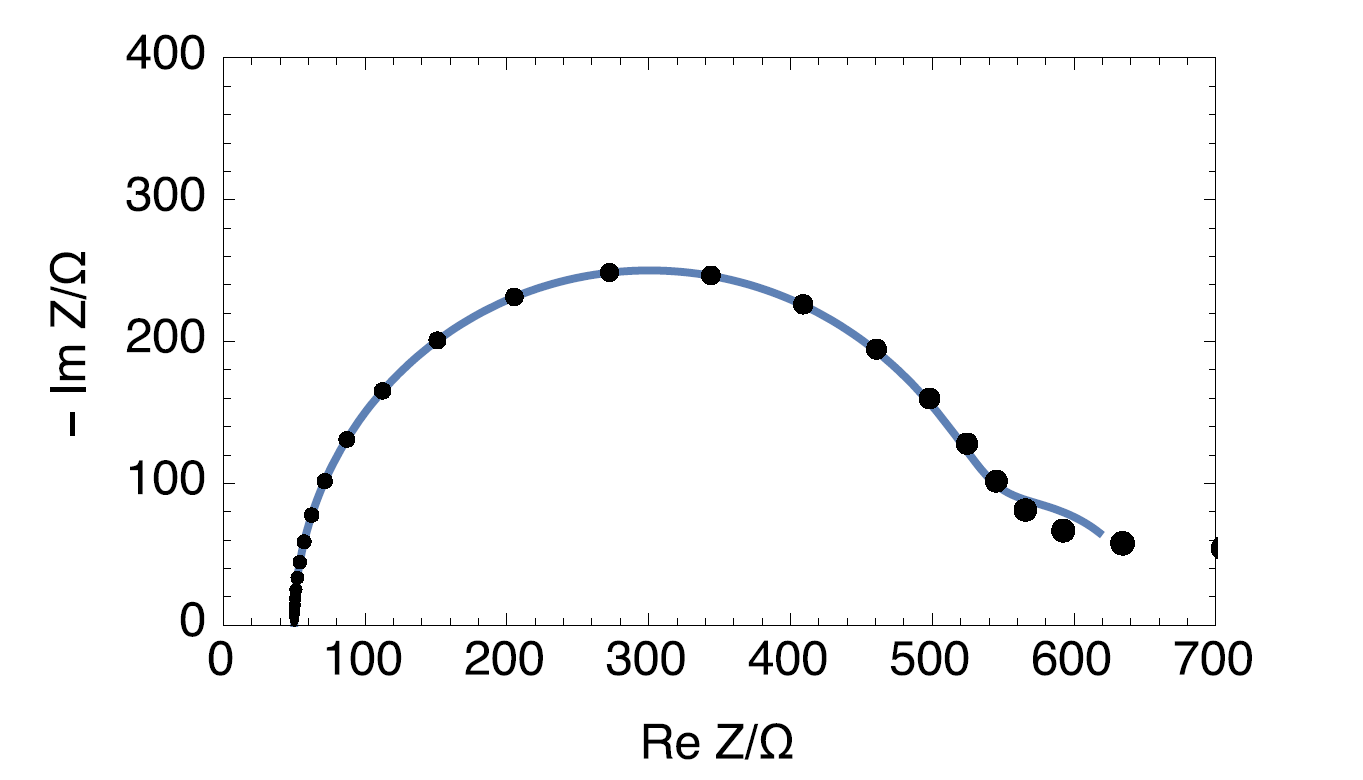
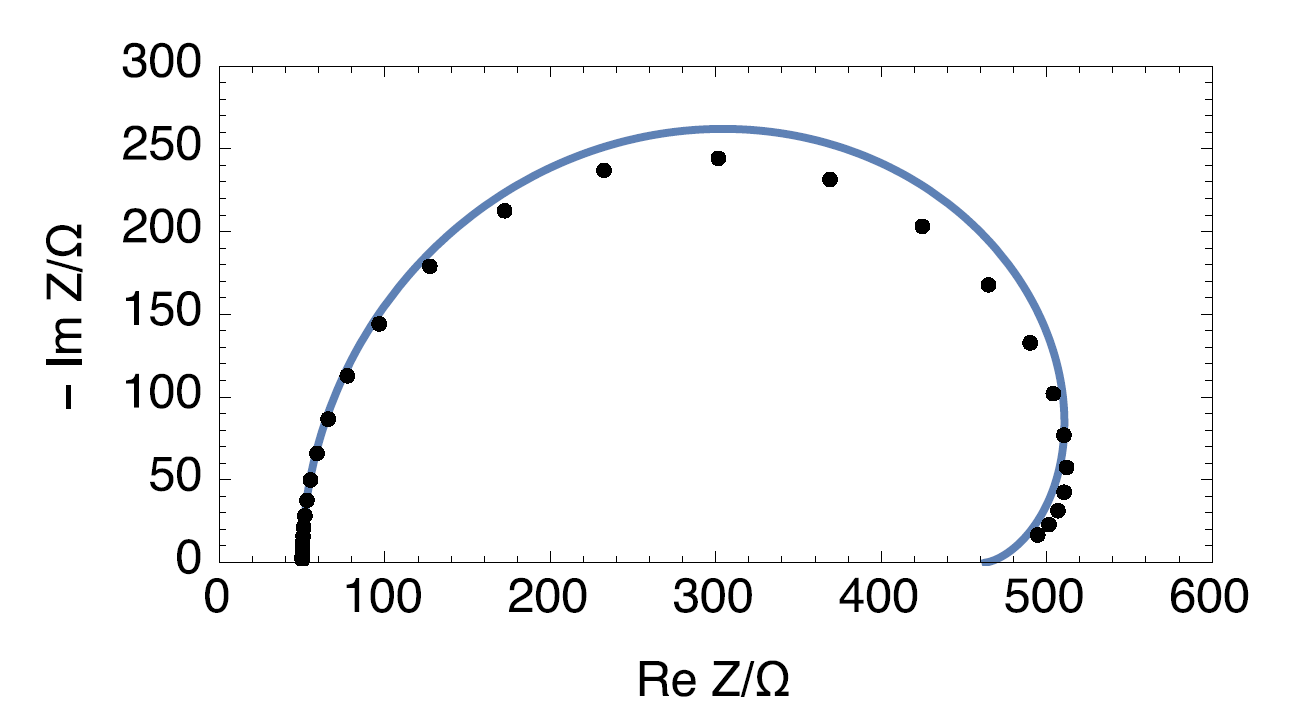
Both graphs are compared in Fig. 5. This shows that a user unaware of the time-variance of the system can mistakenly try to fit the data with a more complicated equivalent circuit than the one required.

Figure 5: Nyquist impedance diagram of the time-variant circuit R1+R2(t)/C2 (•) and Nyquist impedance diagram of the time-invariant circuit R1+C2/(R2+C3/R3), with R1 = 50 Ω, R2 = 500 Ω, C2 = 102 F, R3 = 100 Ω, C3 = 1 F.
R1+R2(t)/C2 Circuit with Decreasing R2
A second case of variation of the resistance R2 is shown in Fig. 6. The value of resistor R2 is henceforth supposed to decrease with time according to
$$R2(t)=R20-k’\sqrt{t}\tag{3}$$

Figure 6: Instantaneous Nyquist impedance diagrams (t = 0 and t = tmax) of the circuit time-variant R1+R2(t)/C2 and simulation of the measured diagram (•).

Figure 7: Nyquist impedance diagram of the time-variant circuit R1+R2(t)/C2 (•) and Nyquist impedance diagram of the time-invariant circuit R1+C2/(R2+C3/R3), with R1 = 50 Ω, R2 = 534.375 Ω, C2 = 1.1.10 2 F, R3 = 123.75 Ω, C3 = 0.125 F.
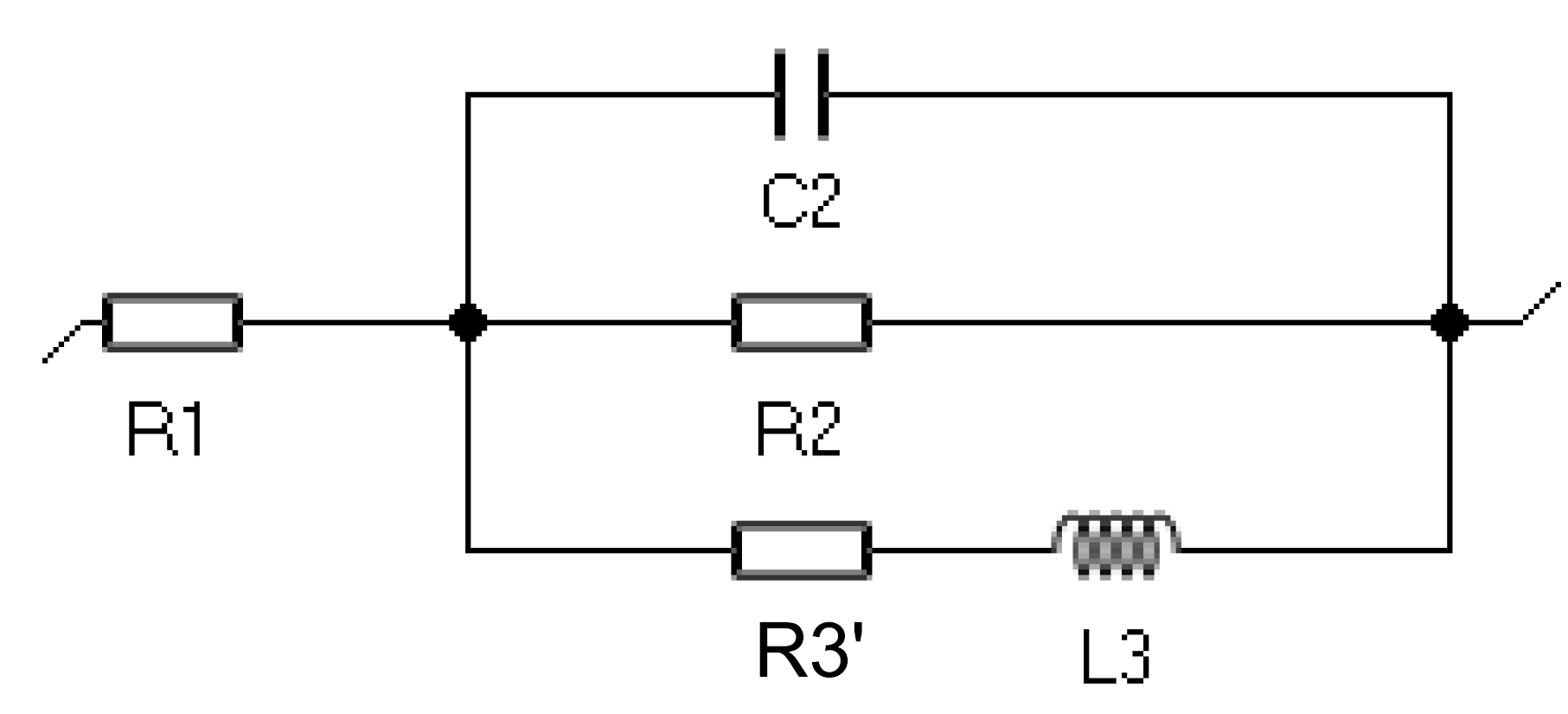
Remember that it is possible to convert the circuit shown in Fig. 4 with R3 < 0 and C3 < 0 to the circuit R1+C2/R2/(R3’+L3) shown in Fig. 8 with R3 > 0 and L3 > 0 where [4]
$$R3’=-\frac{R2(R2+R3)}{R3},L3=-R2^2C3\tag{4}$$

Figure 8: Circuit R1+C2/R2(R3’+L3).
Stationary System Check
Kramers-Kronig (KK) Transforms
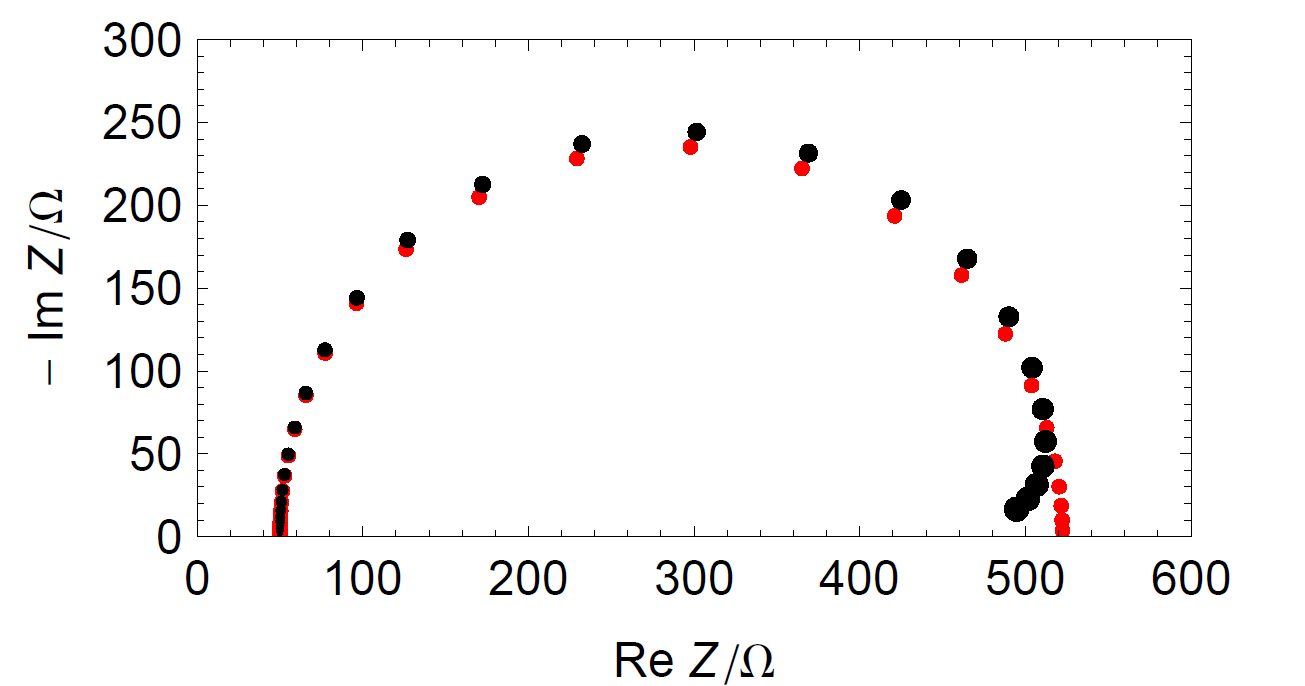
Calculated impedance ZKK using KK transforms is shown in Fig. 9. Nyquist impedance Z and ZKK diagrams are not similar for all frequencies, therefore impedance measurement has not been carried out for a time invariant system [5].

Figure 9: Simulation of the measured Nyquist diagram of the R1+R2(t)/C2 circuit (•) and Nyquist impedance diagram obtained using KK transform (•).
Successive Measurements
A simpler method is to successively perform two identical measurements (Fig. 10). One trick is to successively link two impedance measurements by decreasing frequency fmax fmin then immediately by increasing frequency fmin fmax (Fig. 11).

Figure 10: Simulated Nyquist impedance diagram of the circuit R1+R2(t)/C2 for two successive impedance measurements with scan fmax → fmin.

Figure 11: Simulated Nyquist impedance diagram of the circuit R1+R2(t)/C2 and for two successive impedance measurements with scan fmax → fmin → fmax.
The two impedance diagrams shown in Figs. 10 and 11 are different, which proves that the studied system is time variant.
Conclusion
It is essential to check the stationarity of the system studied by EIS before any interpretation of experimental results. When an electrochemical system changes with time the complexity of the impedance diagram increases and the electrical circuit chosen to interpret the experimental results may be unnecessarily complex.
References
1) Application note #9 “Linear vs. non linear systems in impedance measurements.”
2) Z. Stoynov and B. Savova-Stoynov, J. Elec-troanal. Chem. 183 (1985) 133.
3) F. Berthier, J.-P. Diard, A. Jussiaume, and J.-J. Rameau, Corrosion Science 30 (1990) 239.
4) J.-P. Diard, B. Le Gorrec, and C. Montella, Cinetique électrochimique, Hermann, Paris, (1996).
5) Application note #15 “Two questions about Kramers-Kronig transformations.”
Revised in 08/2019